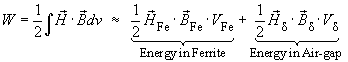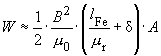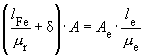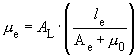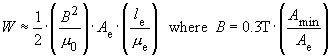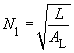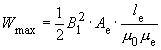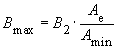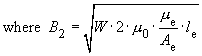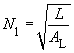Choking Coil

Main page |

Use of the tables |

Calculations |

Tips |

Mathematics used in the program |

Literature Notes

The energy which has to be stored amounts to:

The values L and I_max have been determined on the simulation side.
In order to choose a suitable core, the following requirements for the core must be considered,

• that the magnetic energy storage capacity is at least as big as the above calculated energy ½ ·L I²_max and
• that the core is as small as possible, so that it is inexpensive.

The core table for the selection of suitable cores includes 11 columns, and 12 for the flyback transformer. These are:

• NO.: current number to the list of different cores

• Core: Core type
• Identification: Further identification-charictoristics, e.g. air-gap, material or order code
• Manufacturer: Manufacturer of the core, so more information about the core can be requested if required

• AL/NH: The magnetic conductance. With this one can calculate the number of turns needed for L.
• Ae/mm²: The effective magnetic cross section of the core.
• le/mm: The effective magnetic length of the core.
• Amin/mm²: Minimal core cross-section to calculate the maximum magnetic flux-density.

• Wmax/mWs: The magnetic energy storage capacity of the core, for a maximum magnetic flux density of 0.3T
• Bmax/mT: The maximum magnetic flux density in the core. This magnetic flux density is calculated for the worst case scenario within the input voltage range V_in and nominal load. It is calculated for the minimal cross-section area of the core A_min. The maximum flux density is given as an additional important piece of information in order to help the user to select a suitable core. In order to influence B_max you can change L or ΔI_L on the simulation-side.
• N1: The number of turns for the required inductance L, as well as for L₁ of the flyback converter.
• N2: The secondary number of turns required for the flyback converter. This column only appears if a flyback converter is calculated. To change the secondary number of turns you can change the ratio N₁/N₂ on the simulation side. If the ratio is changed it will influence the maximum drain-source voltage of the transistor. The lower the ratio N₁/N₂ the smaller the drain-source voltage of the transistor V_{ds_max} will be.

The program suggests suitable cores:

• Green writing: Very well-suited cores, whose magnetic energy storage capacity W_max exceeds the required value by a marginal quantity and also has the smallest possible core-volume. The maximum flux-density in these cores approximately reach the saturation point of 0.3T.
• Brown writing: Well suited cores, whose magnetic energy storage capacity clearly lies over the required value. Its core-volume is up to twice as large as the smallest very-well suited core. The maximum flux density for these cores usually lie between 0.2...0.25T.
• Black writing: Suitable cores, whose magnetic energy storage capacity lie very far over the required value. These cores are uneconomically large.
• Gray writing: Inappropriate cores. Under the condition that B_max < 0.3T at every point in the core, the magnetic energy storage capacity lies under the necessary value. The column "Bmax/mT" gives information about the actual maximum flux density. If the core material chosen by you has a higher flux density capibility, you can use this core at your own discretion.

Cores can also be added:
Under the core table are seven input-fields. The fields 'core', 'ID' and 'manufacturer', all serve for the identification of the core and are irrelevant for calculations in the program. The fields 'Al', 'Ae', 'le', and 'Amin' must be filled corresponding to the data sheet. To complete your input click "ADD". The inputed core will be added to the table and treated in the same way as the rest of the pre-determined cores in the table.

Note:
The Wire-diameters proposed by us as well as the Wire-cross-section is always for a declared current density of 3A/mm².

Calculation of choking-coils and flyback transformers

Choking-coils shall store energy. The stored energy amounts to: W = ½ L I ²max. This energy is stored in the form of magnetic field energy. In fact, as well as being stored in the ferrite it is also stored in the air-gap of the core (see right of illustration).

• The required physical size of a choking-coil is approximately proportional to the amount of energy to be stored.

(1)

(2)

• This leads to the following: Choking coils need an air-gap. The energy is stored within this gap.

• Therefore it follows that: The bigger the air-gap the larger the magnetic energy storage capacity.

• effective magnetic core-cross-section A_e,
• the effective magnetic core-length l_e and
• the effective permeability μ_e.

The value μ_e can be calculated by means of magnetic conductance A_L:

The magnetic energy storage capacity then amounts to:

Therefore from the table-values A_e, l_e, A_L and A_min, our program first calculates the magnetic energy storage capacity, and from the result of this produces suggestions for suitable cores.

The number of turns N₁ are calculated with the help of the magnetic conductance A_L:

Calculation of wire-diameter:

The current density S of the winding can be chosen between 2 and 5 A/mm², (depending on the thermal resistance of the transformer). For this it follows that for the wire-cross-section and the wire-diameter:

Note:
The wire-diameters proposed by us are calculated for a current density of 3A/mm².

Tips

• Don't use cores which are too small (Grey Writing) at first, unless you know what you are doing.
• For high frequencies (>50kHz) and larger current ripples (continuous mode) you should select somewhat larger cores (Brown writing). With these the change in flux-density is smaller and with it the hysteresis losses.
• Choose ΔI_L so that it is not too big. The suggestions proposed by us have adequately small current ripple along with physically small inductor size. With a larger current ripple, the voltage ripple of the output voltage V_out becomes clearly bigger while the physical size of the inductor decreases marginally.

Mathematics used in the program

• A_L is the magnetic conductance
• A_e is the effective core cross section
• l_e is the effective core-length
• A_min is the minimum core-cross-section for calculating the maximum magnetic flux density

• W_max: The maximum magnetic energy storage capacity of the core
  W_max is the amount of energy that a core can handle, if the maximum flux-density in the minimal cross-section A_min is exactly B = 0.3T.

  
  
  
  
  For the core selection W_max must be larger than W = ½ L I². A core is economically favorable if it can handle the necessary energy and also have a volume which is as small as possible. According to their volumes A_e·l_e cores are marked with a colour:

  • Cores which are too small (where B in A_min would exceed 0.3T) are written in bright-grey.
  • Cores, whose effective volumes are as small as possible are written in green.
  • Cores that lie 50 to 100% over the smallest volume, are written in brown.
  • Cores, which are even bigger (uneconomically big) are written in black.
• B_max: Maximum flux-density, in the smallest core-cross-section A_min.
  It amounts to:

  
  
  
  
  

• N₁: The number of turns of the inductor or of the flyback converter primary coil.
  The number of turns N₁ amounts to:

  
  
  N₂: Secondary number of turns of the flyback converter transformer.
  N₂ is calculated by using the chosen turns ratio N₁/N₂:

  

Main page |

Use of the tables |

Calculations |

Tips |

Mathematics used in the program |

Literature Notes |

Top of page